Quick Calculation
Length (in feet) x Width (in feet) x Depth (in feet) ÷ 27 = Number of Cubic Yards To Order
Calculation Help
First, all units of measure must be converted to feet. If you are measuring in both feet and inches, convert the inches using this formula:
Inches ÷ 12 (Example: 6 inches ÷ 12 = 0.5 feet)
Calculate Cubic Yards Of Topsoil To Order
STEP 1:
Measure length and width of area to be covered and multiply these numbers together.
TIP: See examples below if you have an irregular area to measure before proceeding to STEP 2.
Length x Width (Example: 20 ft x 10 ft = 200 square feet)
STEP 2:
Multiply your previous answer with the thickness (depth) of soil you want added. Since this is often in inches, you will have to use the conversion to feet shown above. So 10 inches = 0.833 feet.
Previous Answer x Depth (200 sq ft x 0.8333 ft = 166.66 cubic feet)
STEP 3:
Convert cubic feet answer shown above to cubic yards
Previous Answer ÷ 27 (Example 166.66 cu ft ÷ 27 = 6.2 cubic yards to order)
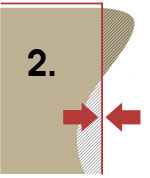
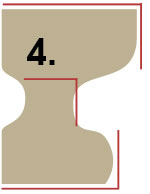
TIP: Calculating Irregular Areas
If your area is not square use the following guidelines to complete STEP 1 above. These may not offer perfect calculations but will give you a more accurate cubic yard measurement when ordering topsoil.
|
Square Area Calculation |
|
|
Curved Side Area |
|
|
Joined Shapes Areas |
|
|
Odd Shape Areas |
 |